Refs:
We need the Axiom LinearOperator library. fricas (1) -> )library CARTEN ARITY CMONAL CPROP CLOP CALEY Use the following macros for convenient notation fricas -- summation macro Σ(x, Type: Void
fricas -- list macro Ξ(f, Type: Void
fricas -- subscript and superscripts macro sb == subscript Type: Void
fricas macro sp == superscript Type: Void
𝐋 is the domain of 2-dimensional linear operators over the rational functions ℚ (Expression Integer), i.e. ratio of polynomials with integer coefficients. fricas dim:=2
Type: PositiveInteger?
fricas macro ℒ == List Type: Void
fricas macro ℂ == CaleyDickson Type: Void
fricas macro ℚ == Expression Integer Type: Void
fricas 𝐋 := ClosedLinearOperator(OVAR ['1,
Type: Type
fricas 𝐞:ℒ 𝐋 := basisOut()
fricas 𝐝:ℒ 𝐋 := basisIn()
fricas I:𝐋:=[1] -- identity for composition
fricas X:𝐋:=[2,
fricas V:𝐋:=ev(1) -- evaluation
fricas Λ:𝐋:=co(1) -- co-evaluation
fricas !:𝐋:=[-1] -- color change 1 -> 1*
fricas $:𝐋:=dagger ! -- 1* -> 1
fricas J:𝐋:=$/!
fricas test(!/$=I)
Type: Boolean
fricas equate(eq)==map((x, Type: Void
We want to be able to implement linear operators with two "colors" like the following:
An example starting with Complex Algebra The basis consists of the real and imaginary units. We use complex multiplication to form the "multiplication table" as a matrix. Then the structure constants can be obtained by dividing each matrix entry by the list of basis vectors. Split-complex can be specified by Caley-Dickson parameter (q0 = -1) fricas --q:=1 -- split-complex
q:=sp('i,
Type: Symbol
fricas QQ := ℂ(ℚ, Type: Type
Basis: Each B.i is a complex number fricas B:ℒ QQ := map(x +-> hyper x,
fricas -- Multiplication table: M:Matrix QQ := matrix Ξ(Ξ(B.i*B.j,
fricas -- Function to divide the matrix entries by a basis element S(y) == map(x +-> real real(x/y), Type: Void
fricas -- The result is a nested list ѕ :=map(S, fricas Compiling function S with type CaleyDickson(Expression(Integer),
Type: List(List(List(Expression(Integer))))
fricas -- structure constants form a tensor operator Y := Σ(Σ(Σ(ѕ(i)(k)(j)*𝐞.i*𝐝.j*($/𝐝.k),
fricas arity Y
fricas Y! := (I,
fricas matrix Ξ(Ξ((𝐞.i*𝐞.j)/Y!,
Multiplication of arbitrary quaternions fricas a:=Σ(sb('a,
fricas b:=Σ(sb('b,
fricas (a,
Multiplication is Associative fricas test( ( Y! J ) / _ ( Y ) = _ ( I Y ) / _ ( Y! ) )
Type: Boolean
A scalar product is denoted by the (2,0)-tensor
fricas U:=Σ(Σ(script('u,
fricas arity U
Definition 1We say that the scalar product is associative if the tensor equation holds:
Y = Y
U U
In other words, if the (3,0)-tensor:
How should we color this? |
|
|
|
last edited 12 years ago by Bill Page |
(13.0,3.8)
\psline[linewidth=0.04cm](11.4,2.6)(13.0,4.2)
\psline[linewidth=0.04cm](13.0,4.2)(13.4,4.2)
\psline[linewidth=0.04cm](13.4,4.2)(13.4,0.4)
\psline[linewidth=0.04cm](13.0,4.2)(13.0,1.0)
\psline[linewidth=0.04cm](13.4,0.4)(13.0,0.4)
\psline[linewidth=0.04cm](13.0,0.4)(10.4,3.2)
\psline[linewidth=0.04cm](13.4,0.4)(10.8,3.2)
\psline[linewidth=0.04cm](10.4,1.6)(10.4,0.4)
\psline[linewidth=0.04cm](10.8,1.6)(10.8,0.4)
\psline[linewidth=0.04cm](10.4,3.2)(10.4,4.2)
\psline[linewidth=0.04cm](10.8,3.2)(10.8,4.2)
\psline[linewidth=0.04cm](10.8,1.6)(11.4,2.2)
\psline[linewidth=0.04cm](10.4,1.6)(11.2,2.4)
\psline[linewidth=0.04cm](10.4,0.4)(10.8,0.4)
\psline[linewidth=0.03cm](10.4,4.2)(10.8,4.2)
\psline[linewidth=0.03cm](6.8,4.2)(6.8,0.4)
\psline[linewidth=0.03cm](7.1850057,4.2)(7.1850057,0.41349542)
\psline[linewidth=0.03cm](6.8,0.41349542)(7.1850057,0.41349542)
\psline[linewidth=0.03cm](6.8,4.2)(7.2,4.2)
\usefont{T1}{ptm}{m}{n}
\rput(8.315937,2.335){\large \psframebox*[framesep=0, boxsep=false,fillcolor=white] {=}}
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](13.2,4.2)(13.2,0.8)
\psline[linewidth=0.04cm](2.4,2.4)(1.0,3.8)
\psline[linewidth=0.04cm](2.6,2.6)(1.0,4.2)
\psline[linewidth=0.04cm](1.0,4.2)(0.6,4.2)
\psline[linewidth=0.04cm](0.6,4.2)(0.6,0.4)
\psline[linewidth=0.04cm](1.0,4.2)(1.0,1.0)
\psline[linewidth=0.04cm](0.6,0.4)(1.0,0.4)
\psline[linewidth=0.04cm](1.0,0.4)(3.6,3.2)
\psline[linewidth=0.04cm](0.6,0.4)(3.2,3.2)
\psline[linewidth=0.04cm](3.6,1.6)(3.6,0.4)
\psline[linewidth=0.04cm](3.2,1.6)(3.2,0.4)
\psline[linewidth=0.04cm](3.6,3.2)(3.6,4.2)
\psline[linewidth=0.04cm](3.2,3.2)(3.2,4.2)
\psline[linewidth=0.04cm](3.2,1.6)(2.6,2.2)
\psline[linewidth=0.04cm](3.6,1.6)(2.8,2.4)
\psline[linewidth=0.04cm](3.6,0.4)(3.2,0.4)
\psline[linewidth=0.03cm](3.6,4.2)(3.2,4.2)
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](0.8,4.2)(0.8,0.8)
\usefont{T1}{ptm}{m}{n}
\rput(5.5159373,2.335){\large \psframebox*[framesep=0, boxsep=false,fillcolor=white] {=}}
\psline[linewidth=0.03cm,linestyle=dotted,dotsep=0.16cm](0.0,1.6)(14.0,1.6)
\psline[linewidth=0.03cm,linestyle=dotted,dotsep=0.16cm](0.0,3.2)(14.0,3.2)
\usefont{T1}{ptm}{m}{n}
\rput(6.9159374,-2.265){\large \psframebox*[framesep=0, boxsep=false,fillcolor=white] {=}}
\psline[linewidth=0.04cm](4.2,-1.4)(3.2,-0.4)
\psline[linewidth=0.04cm](4.6,-1.4)(3.6,-0.4)
\psline[linewidth=0.04cm](3.6,-0.4)(3.2,-0.4)
\psline[linewidth=0.04cm](3.2,-0.4)(3.2,-4.2)
\psline[linewidth=0.04cm](3.6,-0.8)(3.6,-3.6)
\psline[linewidth=0.04cm](3.2,-4.2)(3.6,-4.2)
\psline[linewidth=0.04cm](3.6,-4.2)(4.6,-3.0)
\psline[linewidth=0.04cm](3.2,-4.2)(4.2,-3.0)
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](3.4,-0.8)(3.4,-4.0)
\psline[linewidth=0.03cm](4.2,-1.4)(4.2,-3.0)
\psline[linewidth=0.03cm](4.6,-1.4)(4.6,-3.0)
\psline[linewidth=0.04cm](9.6,-1.4)(10.6,-0.4)
\psline[linewidth=0.04cm](9.2,-1.4)(10.2,-0.4)
\psline[linewidth=0.04cm](10.2,-0.4)(10.6,-0.4)
\psline[linewidth=0.04cm](10.6,-0.4)(10.6,-4.2)
\psline[linewidth=0.04cm](10.2,-0.8)(10.2,-3.6)
\psline[linewidth=0.04cm](10.6,-4.2)(10.2,-4.2)
\psline[linewidth=0.04cm](10.2,-4.2)(9.2,-3.0)
\psline[linewidth=0.04cm](10.6,-4.2)(9.6,-3.0)
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](10.4,-0.8)(10.4,-4.0)
\psline[linewidth=0.03cm](9.6,-1.4)(9.6,-3.0)
\psline[linewidth=0.03cm](9.2,-1.4)(9.2,-3.0)
\end{pspicture}
}
\scalebox{1} % Change this value to rescale the drawing.
{
\begin{pspicture}(0,-4.22)(14.015,4.22)
\psline[linewidth=0.04cm](11.6,2.4)(13.0,3.8)
\psline[linewidth=0.04cm](11.4,2.6)(13.0,4.2)
\psline[linewidth=0.04cm](13.0,4.2)(13.4,4.2)
\psline[linewidth=0.04cm](13.4,4.2)(13.4,0.4)
\psline[linewidth=0.04cm](13.0,4.2)(13.0,1.0)
\psline[linewidth=0.04cm](13.4,0.4)(13.0,0.4)
\psline[linewidth=0.04cm](13.0,0.4)(10.4,3.2)
\psline[linewidth=0.04cm](13.4,0.4)(10.8,3.2)
\psline[linewidth=0.04cm](10.4,1.6)(10.4,0.4)
\psline[linewidth=0.04cm](10.8,1.6)(10.8,0.4)
\psline[linewidth=0.04cm](10.4,3.2)(10.4,4.2)
\psline[linewidth=0.04cm](10.8,3.2)(10.8,4.2)
\psline[linewidth=0.04cm](10.8,1.6)(11.4,2.2)
\psline[linewidth=0.04cm](10.4,1.6)(11.2,2.4)
\psline[linewidth=0.04cm](10.4,0.4)(10.8,0.4)
\psline[linewidth=0.03cm](10.4,4.2)(10.8,4.2)
\psline[linewidth=0.03cm](6.8,4.2)(6.8,0.4)
\psline[linewidth=0.03cm](7.1850057,4.2)(7.1850057,0.41349542)
\psline[linewidth=0.03cm](6.8,0.41349542)(7.1850057,0.41349542)
\psline[linewidth=0.03cm](6.8,4.2)(7.2,4.2)
\usefont{T1}{ptm}{m}{n}
\rput(8.315937,2.335){\large \psframebox*[framesep=0, boxsep=false,fillcolor=white] {=}}
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](13.2,4.2)(13.2,0.8)
\psline[linewidth=0.04cm](2.4,2.4)(1.0,3.8)
\psline[linewidth=0.04cm](2.6,2.6)(1.0,4.2)
\psline[linewidth=0.04cm](1.0,4.2)(0.6,4.2)
\psline[linewidth=0.04cm](0.6,4.2)(0.6,0.4)
\psline[linewidth=0.04cm](1.0,4.2)(1.0,1.0)
\psline[linewidth=0.04cm](0.6,0.4)(1.0,0.4)
\psline[linewidth=0.04cm](1.0,0.4)(3.6,3.2)
\psline[linewidth=0.04cm](0.6,0.4)(3.2,3.2)
\psline[linewidth=0.04cm](3.6,1.6)(3.6,0.4)
\psline[linewidth=0.04cm](3.2,1.6)(3.2,0.4)
\psline[linewidth=0.04cm](3.6,3.2)(3.6,4.2)
\psline[linewidth=0.04cm](3.2,3.2)(3.2,4.2)
\psline[linewidth=0.04cm](3.2,1.6)(2.6,2.2)
\psline[linewidth=0.04cm](3.6,1.6)(2.8,2.4)
\psline[linewidth=0.04cm](3.6,0.4)(3.2,0.4)
\psline[linewidth=0.03cm](3.6,4.2)(3.2,4.2)
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](0.8,4.2)(0.8,0.8)
\usefont{T1}{ptm}{m}{n}
\rput(5.5159373,2.335){\large \psframebox*[framesep=0, boxsep=false,fillcolor=white] {=}}
\psline[linewidth=0.03cm,linestyle=dotted,dotsep=0.16cm](0.0,1.6)(14.0,1.6)
\psline[linewidth=0.03cm,linestyle=dotted,dotsep=0.16cm](0.0,3.2)(14.0,3.2)
\usefont{T1}{ptm}{m}{n}
\rput(6.9159374,-2.265){\large \psframebox*[framesep=0, boxsep=false,fillcolor=white] {=}}
\psline[linewidth=0.04cm](4.2,-1.4)(3.2,-0.4)
\psline[linewidth=0.04cm](4.6,-1.4)(3.6,-0.4)
\psline[linewidth=0.04cm](3.6,-0.4)(3.2,-0.4)
\psline[linewidth=0.04cm](3.2,-0.4)(3.2,-4.2)
\psline[linewidth=0.04cm](3.6,-0.8)(3.6,-3.6)
\psline[linewidth=0.04cm](3.2,-4.2)(3.6,-4.2)
\psline[linewidth=0.04cm](3.6,-4.2)(4.6,-3.0)
\psline[linewidth=0.04cm](3.2,-4.2)(4.2,-3.0)
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](3.4,-0.8)(3.4,-4.0)
\psline[linewidth=0.03cm](4.2,-1.4)(4.2,-3.0)
\psline[linewidth=0.03cm](4.6,-1.4)(4.6,-3.0)
\psline[linewidth=0.04cm](9.6,-1.4)(10.6,-0.4)
\psline[linewidth=0.04cm](9.2,-1.4)(10.2,-0.4)
\psline[linewidth=0.04cm](10.2,-0.4)(10.6,-0.4)
\psline[linewidth=0.04cm](10.6,-0.4)(10.6,-4.2)
\psline[linewidth=0.04cm](10.2,-0.8)(10.2,-3.6)
\psline[linewidth=0.04cm](10.6,-4.2)(10.2,-4.2)
\psline[linewidth=0.04cm](10.2,-4.2)(9.2,-3.0)
\psline[linewidth=0.04cm](10.6,-4.2)(9.6,-3.0)
\psline[linewidth=0.03cm,linestyle=dashed,dash=0.16cm 0.16cm](10.4,-0.8)(10.4,-4.0)
\psline[linewidth=0.03cm](9.6,-1.4)(9.6,-3.0)
\psline[linewidth=0.03cm](9.2,-1.4)(9.2,-3.0)
\end{pspicture}
}](images/8979932646826338497-16.0px.png)
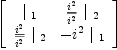
(2.2,0.1)(2.6,0.1)(2.6,0.9)
\psline[linewidth=0.04cm](2.4,0.3)(2.4,-0.1)
\psbezier[linewidth=0.04](2.4,-0.1)(2.4,-0.9)(3.0,-0.9)(3.0,-0.1)
\psline[linewidth=0.04cm](3.0,-0.1)(3.0,0.9)
\psbezier[linewidth=0.04](4.8,0.9)(4.8,0.1)(4.4,0.1)(4.4,0.9)
\psline[linewidth=0.04cm](4.6,0.3)(4.6,-0.1)
\psbezier[linewidth=0.04](4.6,-0.1)(4.6,-0.9)(4.0,-0.9)(4.0,-0.1)
\psline[linewidth=0.04cm](4.0,-0.1)(4.0,0.9)
\usefont{T1}{ptm}{m}{n}
\rput(3.4948437,0.205){-}
\psline[linewidth=0.04cm](0.6,-0.7)(0.6,0.9)
\psbezier[linewidth=0.04](0.0,-0.1)(0.0,-0.9)(1.2,-0.9)(1.2,-0.1)
\psline[linewidth=0.04cm](0.0,-0.1)(0.0,0.9)
\psline[linewidth=0.04cm](1.2,-0.1)(1.2,0.9)
\usefont{T1}{ptm}{m}{n}
\rput(1.6948438,0.205){=}
\end{pspicture}
}
\scalebox{1} % Change this value to rescale the drawing.
{
\begin{pspicture}(0,-0.92)(4.82,0.92)
\psbezier[linewidth=0.04](2.2,0.9)(2.2,0.1)(2.6,0.1)(2.6,0.9)
\psline[linewidth=0.04cm](2.4,0.3)(2.4,-0.1)
\psbezier[linewidth=0.04](2.4,-0.1)(2.4,-0.9)(3.0,-0.9)(3.0,-0.1)
\psline[linewidth=0.04cm](3.0,-0.1)(3.0,0.9)
\psbezier[linewidth=0.04](4.8,0.9)(4.8,0.1)(4.4,0.1)(4.4,0.9)
\psline[linewidth=0.04cm](4.6,0.3)(4.6,-0.1)
\psbezier[linewidth=0.04](4.6,-0.1)(4.6,-0.9)(4.0,-0.9)(4.0,-0.1)
\psline[linewidth=0.04cm](4.0,-0.1)(4.0,0.9)
\usefont{T1}{ptm}{m}{n}
\rput(3.4948437,0.205){-}
\psline[linewidth=0.04cm](0.6,-0.7)(0.6,0.9)
\psbezier[linewidth=0.04](0.0,-0.1)(0.0,-0.9)(1.2,-0.9)(1.2,-0.1)
\psline[linewidth=0.04cm](0.0,-0.1)(0.0,0.9)
\psline[linewidth=0.04cm](1.2,-0.1)(1.2,0.9)
\usefont{T1}{ptm}{m}{n}
\rput(1.6948438,0.205){=}
\end{pspicture}
}](images/2375189246716000159-16.0px.png)